ENGR2000 Lecture Notes - Lecture 9: Surface Tension, Gravity Bone, John Wiley & Sons

Prepared by Dr Hongwei Wu Chapter 9 – Page 1 of 13
2nd – Year Fluid Mechanics, Faculty of Engineering and Computing, Curtin University
ENGR2000: FLUID MECHANICS
For Second-Year Chemical, Civil and Mechanical Engineering
FLUID MECHANICS LECTURE NOTES
CHAPTER 9 MODELS, DIMENSIONAL ANALYSIS AND SIMILITUDE
In Chapter 6, we have shown that some of the practical engineering problems can be solved
by theoretical analysis, for example, the calculation of the pressure drop of a laminar pipe
flow. However, there are still a large number (in fact, most) of real fluid problems cannot be
solved by theoretical analysis alone, for example, the pressure drop of a turbulent pipe flow.
Solutions of such problems have to rely on experimental investigations, or at least a
combination of theoretical and experimental investigations. This chapter introduces several
important concepts in fluid mechanics, including model, dimensional analysis and similitude.
These concepts are of critical importance to smart experimental planning and design to obtain,
understand and correlate quality experimental data, producing results that can be widely used.
9.1 Model
9.1.1 What is a model?
A model is an intellectual construct that has two basic characteristics: 1) representing reality;
2) being able to predict the consequences of future actions.
EX9-1: A simple example
Newton’s 2nd motion law:
onAcceleratiMassForce
i.e.
maF
This law is a simple mathematical model of the relation between force,
mass and acceleration. It indeed has been validated so that we have the
confidence to use it.
There are two types of models: computational model and physical model. A computational
model relies on the simulation using mathematical models while a physical model is based on
a physical representation of prototype by scaling-up or scaling-down. In practice, it is very
difficult to have completely reliable computational models. Critical engineering decisions are
very often made on the basis of a combination of computational and physical models.
Therefore, A model must be validated by experimental observations or data before we can
have enough confidence in using this model.
9.1.2 Why do we need a model?
Before a process can be implemented in real scale applications, we need to be sure that it will
work in the way we wanted. Otherwise, there is significant risk to waste large amount of
money or even claim human lives. It is often said that
“Have your failures on a small scale, in private;
Have your successes on a large scale, in public.” - Quoted from reference [1]
Therefore, model scaling-up (or scaling-down) are essential. Typical engineering examples
include models of new aircrafts, chemical reactors, etc.
find more resources at oneclass.com
find more resources at oneclass.com
Prepared by Dr Hongwei Wu Chapter 9 – Page 2 of 13
9.2 Scaling and the need of dimensionless numbers
9.2.1 Scaling
The proper construction of a model requires the scaling up or down of the prototype.
However, without proper scaling rules, it is NOT guaranteed that the model and the prototype
will have comparable performance. The following is an example from reference [1].
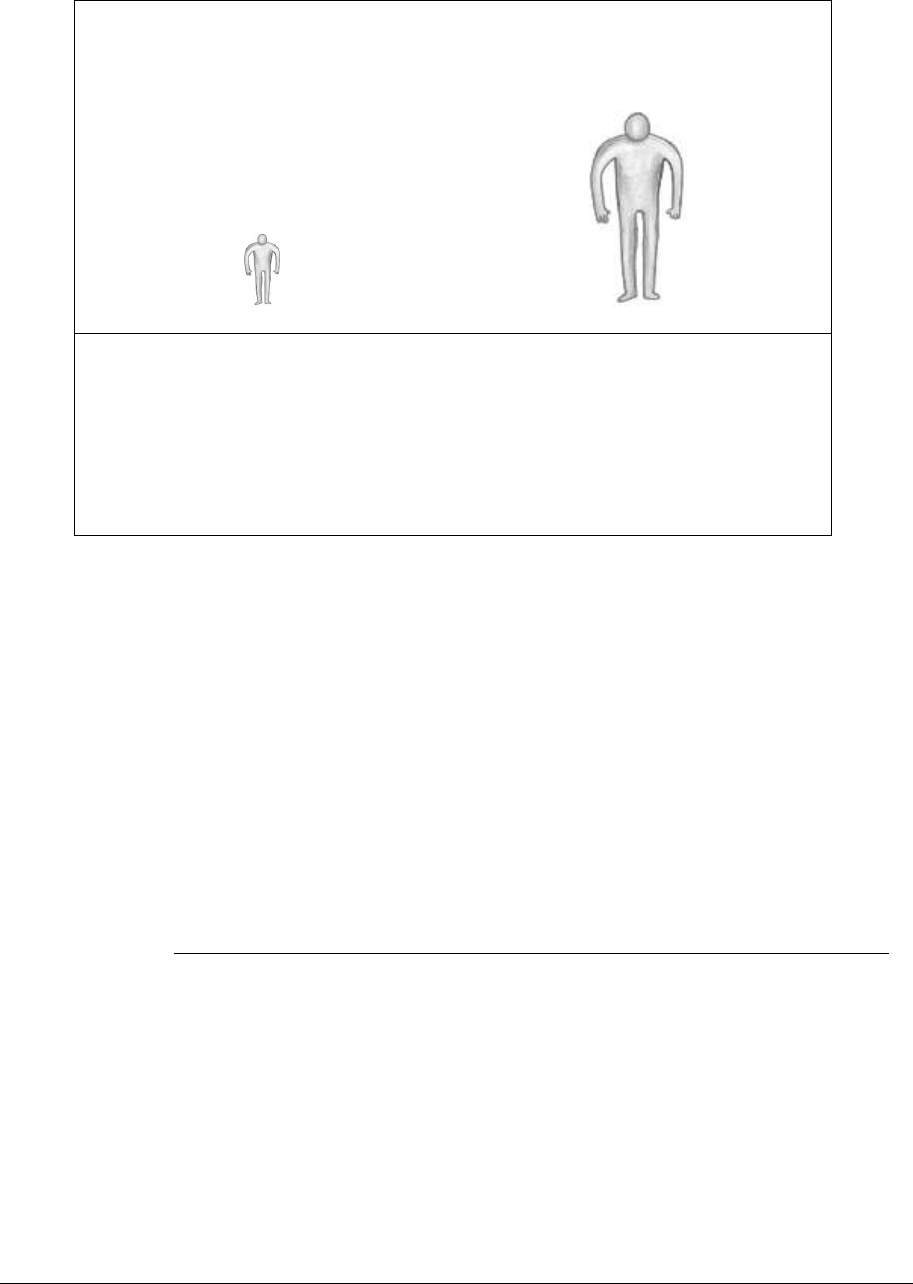
EX9-2: An example – creation of giant human
We assume that the creator has a new task to create giant humans who will
live on the earth. A giant human has not only 10 times as high as a normal
human but also 10 times as wide and 10 times as thick.
A normal human
A giant human
Therefore, a giant human’s weight (W) is about 1000 times the normal
human’s:
normalgiant WW 1000
. However, the cross-section (A) of the
bone of a giant man is only 100 times that of a normal human:
normalgiant AA 100
.
The giant human will NOT be able to stand on the earth!!!
Obviously, holding the shape constant while increasing the size does not guarantee equal
performance by differently sized human, or equipment. To construct a walkable giant human,
the creator would need to do more thinking. In the case of the bones of the giant, one should
keep constant the ratio of the stress and crushing strength, with one of the following choices:
1. As the height of the giant is increased, the crushing strength of the giant’s bones could
be increased.
2. The average density of the giant’s body could be reduced in order to keep the same
bone stress while the height is increased.
3. The giant can live in another planet with a lower acceleration of gravity.
9.2.2 Dimensionless numbers
From the discussion in above section, it is clear that to construct a walkable giant human, the
creator needs to consider a parameter called “Bone Ratio” defined as the following:
bonesofstrengthCrushing
gravityofonAcceleratidensitybodyAverageHeight
RatioBone
If the Bone Ratio is kept as constant, the giant can become any height with the same relative
resistance to bone failure as any normal human has. The bone ratio is dimensionless.
Dimensionless ratios like the Bone Ratio are extremely important in predicting a large piece
of equipment from tests on a small model in engineering practice. Also, such dimensionless
ratios are also valuable in correlating, interpreting, and comparing experimental data. The
following is another simple example.
find more resources at oneclass.com
find more resources at oneclass.com

Prepared by Dr Hongwei Wu Chapter 9 – Page 3 of 13
EX9-3: An example – investment return
Compare a business venture in which you invest $4000 for a return of $400
per year with one in which you invests $6000 for a return of $720 per year.
Which dimensionless number should we use?
The percentage, the most commonly used dimensionless number.
9.2.3 Importance of dimensionless numbers in fluid mechanics
In Fluid Mechanics, dimensionless numbers play very important roles in investigating
physical phenomena, understanding flow behaviours and solving practical engineering
problems. Let’s visit an example.
We know that key factors influencing pressure gradient along a smooth pipe include the pipe
diameter D, the fluid density
, fluid viscosity
, and the mean velocity V at which the fluid is
flowing through the pipe. The pressure gradient
P/
L (
P is the pressure drop across a pipe
with length
L) can then be expressed as a function as
),,,( VDf
L
P
(E9-1)
One may suggest that the easiest way to work out this function is to design sets of
experiments by varying only one of the four variables at a time while keeping the rest three
variables as constant. At first glance, this sounds indeed a good idea because we can foresee
that Figure 9-1a to Figure9-1d may be obtained through these sets of experiments.
Performing experiments to derive Figure 9-1a is easy, which actually was done by Osborne
Reynolds [3] in 1883 (see Region III of Figure 6-3). Performing experiments to derive Figure
9-1b is also possible, although the range of pipe diameter to be tested will be limited due to
engineering constraints. However, carrying out experiments in Figures 9-1c and 9-1d are very
difficult. The reason is that it is practically extremely difficult, if not impossible, to vary fluid
density while still keep fluid viscosity as constant, or vice versa. Actually, even if we are able
to do all experiments in Figures 9-1a to d, we still do not know the exact correlation.
In fact, life would become much easier if we can do a set of experiments to obtain Figure 9-2.
Rather than exhaust our efforts to obtain Figure 9-1, we can simplify the problem into
performing a set of experiments to derive the correlation between two dimensionless
numbers: friction factor (f) and Reynolds number (Re) as a single curve, i.e.
(Re)f
. This
is what have in the Moody chart, i.e. F6-16 or equation E6-26b.
EX9-4: An example – Experiment Design
We have been requested to investigate the pressure gradient (pressure drop
per unit length) along a smooth pipe. We need to decide the key factors
influencing pressure gradient, how to do the experiments, and how to derive
necessary correlations for practical use.
What should we do?
find more resources at oneclass.com
find more resources at oneclass.com
Document Summary
2nd year fluid mechanics, faculty of engineering and computing, curtin university. In chapter 6, we have shown that some of the practical engineering problems can be solved by theoretical analysis, for example, the calculation of the pressure drop of a laminar pipe flow. However, there are still a large number (in fact, most) of real fluid problems cannot be solved by theoretical analysis alone, for example, the pressure drop of a turbulent pipe flow. Solutions of such problems have to rely on experimental investigations, or at least a combination of theoretical and experimental investigations. This chapter introduces several important concepts in fluid mechanics, including model, dimensional analysis and similitude. These concepts are of critical importance to smart experimental planning and design to obtain, understand and correlate quality experimental data, producing results that can be widely used. A model is an intellectual construct that has two basic characteristics: 1) representing reality: being able to predict the consequences of future actions.

