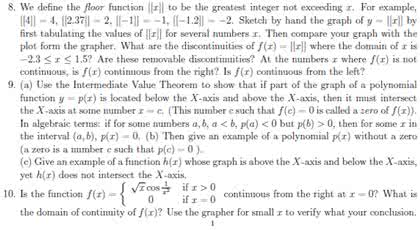
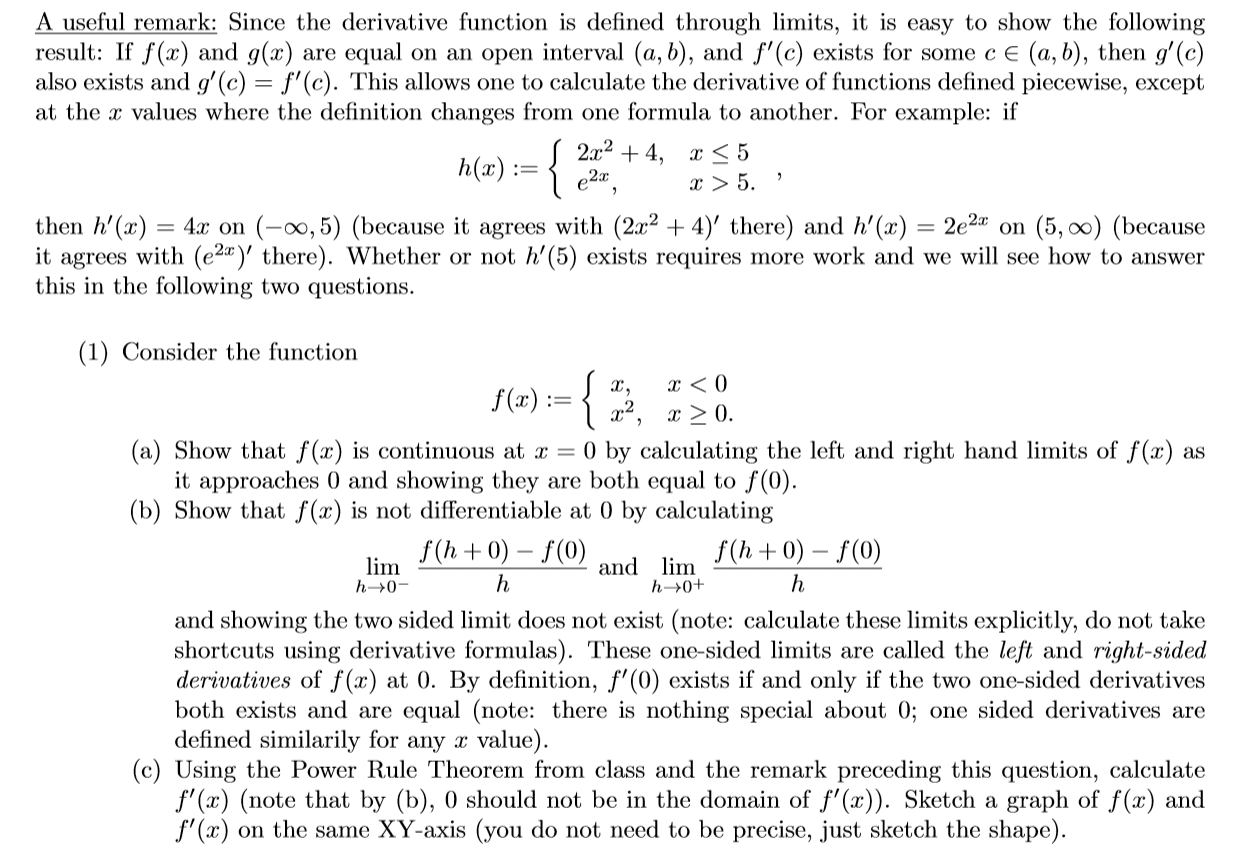
MATH 140 Lecture Notes - Lecture 9: Intermediate Value Theorem, Classification Of Discontinuities, Oliver Heaviside

59
MATH 140 Full Course Notes
Verified Note
59 documents
Document Summary
We noticed in section 2. 3 that the limit of a function as approaches can often be found simply by calculating the value of the function at . Functions with this property are called continuous at a. We will see that the mathematical de nition of continuity corresponds closely with the meaning of the word continuity in everyday language. (a continuous process is one that takes place gradually, without interruption or abrupt change. ) Math140 - lecture 9 notes a a x. Continuity: is continuous at a number a if. The limit of a function as x approaches a can be often found by calculating the value of the function at a . Functions with this property are usually called continuous at a . De nition: a function f is continuous at a number a if: Notice that de nition l implicitly requires three things if f a lim (x > a) f(x) = f(a)