MATH 133 Lecture Notes - Elementary Matrix, Identity Matrix, Invertible Matrix

• School: McGill University
• Department: Mathematics & Statistics (Sci)
• Course: MATH 133
• Professor: Djivede Kelome

Document Summary

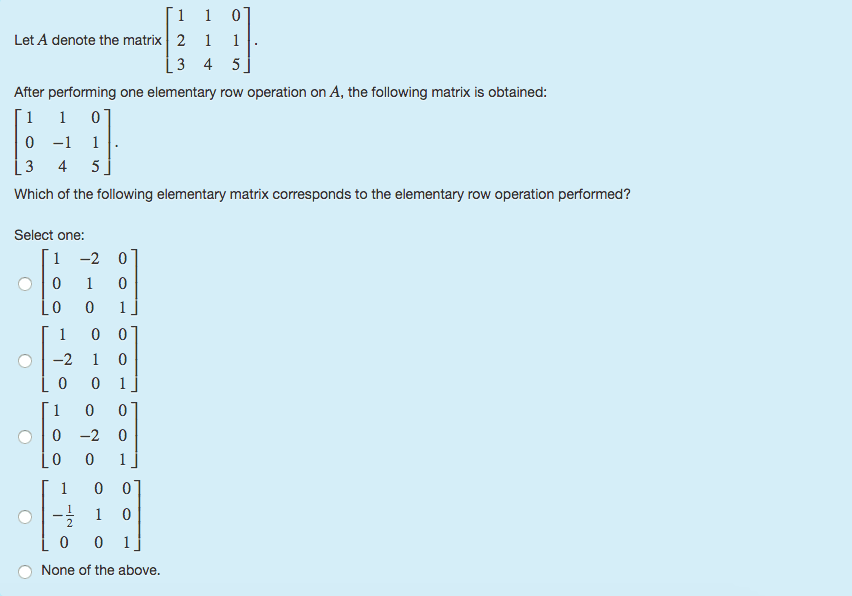
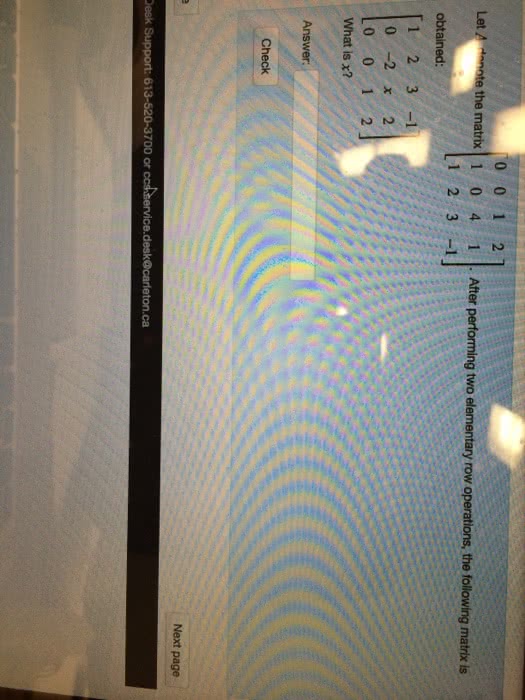
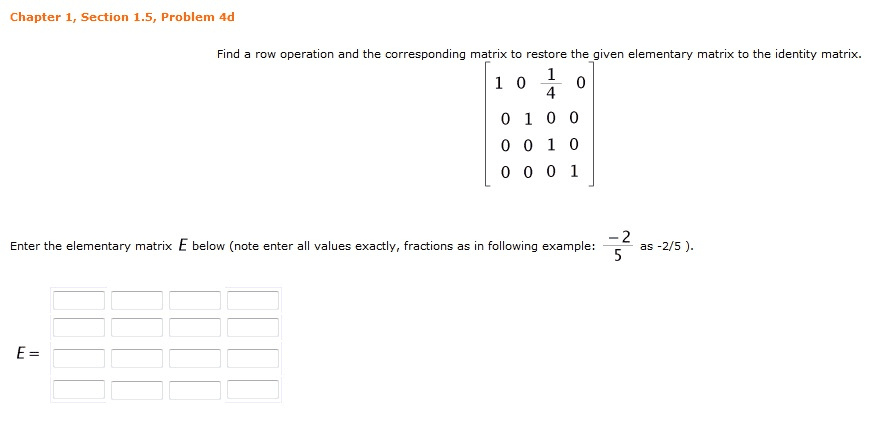
A nxn elementary matrix is a matrix obtained by performing a single elementary row operation ont he nxn identity matrix (in) Note that e is obtained by exchanging r and. E is obtained by multiplying r by 5 starting with. Performing a single row operation on a is equivalent to multiplying. A from the left by an elementary matrix. E; eis obtained by performing the same row operation. Theorem: if e is an elementary matrix then e is invertible and actually. Suppose that e is obtained from by exchanging r and r. Suppose that e. is obtained from by multiplying r by k (k=0) is obtained from. by multiplying r. by. Suppose that e. is obtained from. by replacing r. with r + br. To carry into a rref matrix b we used a sequence of elementary row operations. Theorem: if a is an nxm matrix and b is its corresponding unique.