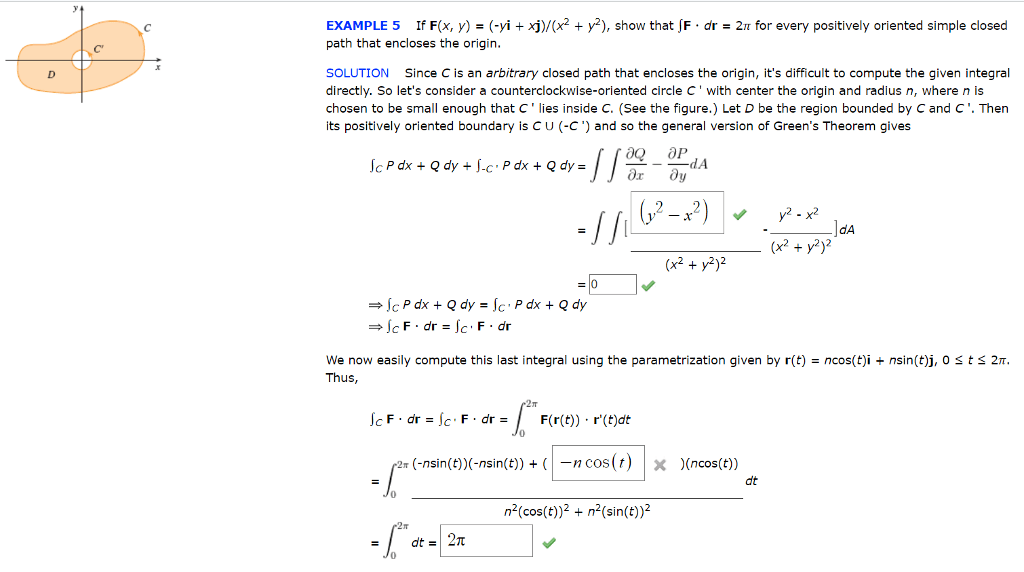
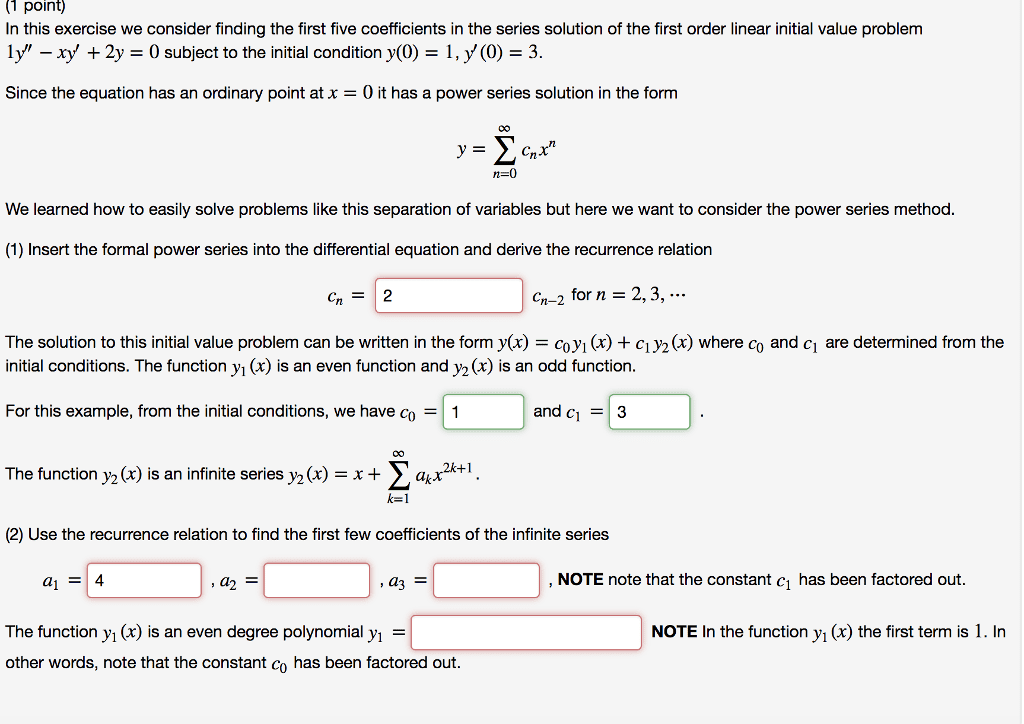
Consider a dilute solution of glucose in water at 25 oC. The solution was in a horizontal tube of length 10 cm, at was prepared such that there was a linear gradation of glucose concentration from cglucose(0, 0) = 0.1M at x = 0 cm to cglucose(0, 10cm) = 0.05M at x = 10 cm. (A) What is the force (and direction) acting at the center of the tube (x = 5 cm)? Derive a formula for cglucose(0, x) for x â [0, 10]cm. (B) We wish to solve the time time dependence of cglucose(t, x) for all times t > 0. The next steps of this question will lead you through how to solve such a problem. Consider Fickâs second law of diffusion in one dimension, âc ât = D â 2 c âx2 (2) Show that a function of the form c(t, x) = exp(λt) à (Aeikx + Beâikx) satisfies Eq. (2) when λ is related to k and D. Determine λ for such a solution. (C) For the problem like that considered in (A) for particles confined to a a region of length L where x â (0, L) (in (A), L = 10 cm, for example) The flux at the end of the containers is equal to zero (no particles leave or enter the container). Mathematically, this imposes a boundary condition that J(x â 0â) = J(x â L+) = 0 (x â 0â means you approach x = 0 from the left and x â L+ means you approach x = L from the right). Show that these boundary conditions imply that A = B and k â¡ kn = nÏ L for n = 0, 1, 2, ...â. That is, show that solutions look like 2Aeλnt cos nÏx L (where we now relate λn to kn). (D) Spatially normalize the solutions. Letâs denote the solutions to Eq. (2) with the above boundary conditions as cn(t, x) = AË ne λnt cos nÏx d for n = 0, 1, 2, ...â. We wish to verify that at t = 0, these solutions are orthonormal. What the means is we want to show: Z L 0 dxcn(0, x)cm(0, x) = 0 (3) for n 6= m and Z L 0 dxc0(0, x)c0(0, x) = L(AË 0) 2 = 1 Z L 0 dxcn6=0(0, x)cn6=0(0, x) = L 2 (AË n) 2 = 1 (4) 3 (Hint: it may be easier to verify the above integrals using the relationships that cos(θ) = e iθ+eâiθ 2 and sin(θ) = e iθâeâiθ 2i ). From the above orthonormality conditions, determine what An for n = 0, 1, 2, ...â. (E) Superposition principle. Verify that c(t, x) = Pâ n=0 bncn(t, x) satisfies Eq. (2) and also the boundary condition that âc(t,x) âx xâ0â = âc(t,x) âx xâL+ = 0. The coefficients, bn are simple numbers telling you how much of cn(t, x) is part of your solution for c(t, x). (F) Imposing the initial condition at t = 0. Suppose that at t = 0, c(0, x) = F +Gx for x â (0, L) and c(0, x) = 0 for x ⤠0 and x ⥠L, i.e., there is a linear gradation to the concentration within the sample region. By the superposition principle, we can write c(t, x) as c(t, x) = X n=0 bncn(t, x) (5) At t = 0 we must have: c(0, x) = F + Gx = X n=0 bncn(0, x) (6) Using orthonormality conditions you derived above, show that bn = Z L 0 dxcn(0, x)(F + Gx) (7) Explicitly determine formulas for b0 and bn6=0. Using these expressions, finally write out your solution to c(t, x). (G) Finally, letâs go back to (A) and determine how the glucose concentration changes with time. The diffusion coefficient for glucose in water is D = 5.7 à 10â10 m2 s . Numerically calculate cglucose(t, x) for x â (0, L) at t = 0.5 s, t = 1s, t = 10s, and t = 1000 s. You will need to approximate your formulas for c(t, x) by taking only a finite number of terms.