MATH136 Lecture Notes - Linear Combination, International Sport Karate Association, Hyperplane

34
MATH136 Full Course Notes
Verified Note
34 documents
Document Summary
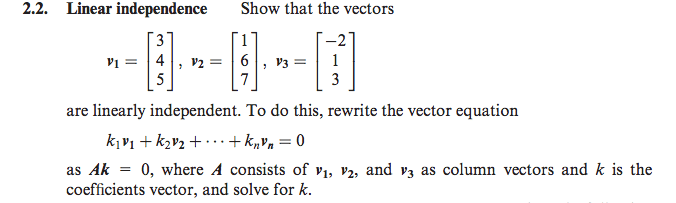
Wednesday, january 8 lecture 2 : linear independence of vectors in n: linearly independent subset in n, a plane in 3. Let v1, v2, , vk be k vectors in n and suppose the vector 0 represents the zero vector. The vectors v1, v2,, vk are said to be linearly independent if the only way that: characterization of a linearly independent set as one being a set where no vector. 2. 1 definitions. is a linear combination of the others. can hold true is if 1, 2, , k are all zeroes. The solution where all the i s are zeros is called the trivial solution of this vector equation. If v1, v2, , vk are not linearly independent then they are said to be linearly dependent. Note that a linearly independent set cannot contain the zero-vector, 0. In class, we will often abbreviate the words linearly independent with the letters l. i.