MATH136 Lecture Notes - Pythagorean Theorem, Linear Algebra, Hypotenuse

• School: University of Waterloo
• Department: Mathematics
• Course: MATH136
• Professor: Robert Andre

Document Summary

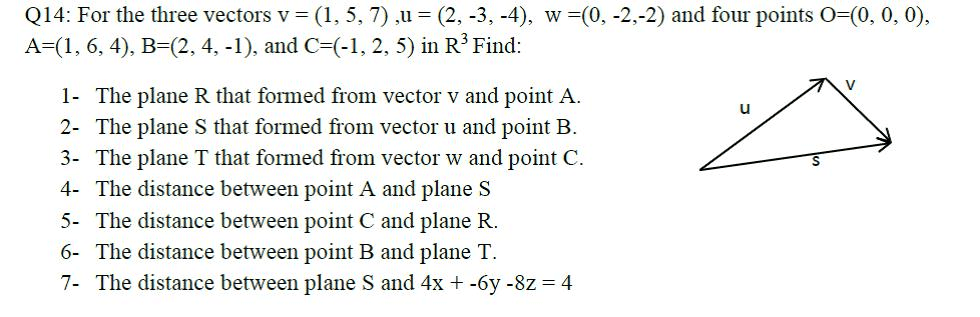
Suppose we are given a vector b in (cid:1337)2 and another vector a. 7. 2 definition (cid:16) let b be a vector in (cid:1337)2 or (cid:1337)3 and let a be any other vector in the same point on the line l = {ta : t (cid:143)(cid:3)(cid:1337)} which is the closest to b. If in (cid:1337)2, we have defined p = proja(b) so that the points (0, 0), p, and b form a right. P is denoted by perpab. (this expression is pronounced perpab onto a ). So vector p = proja(b) is a scalar multiple of the directed line segment a. space. 7. 1 projections (cid:16) occasionally, one might want to express a vector x as a sum of two orthogonal vectors (perpendicular vectors), called orthogonal component vectors of x. triangle. (the directed line segment of b forming the hypotenuse). So it is always true that (cid:32) proja(b) + perpa(b)