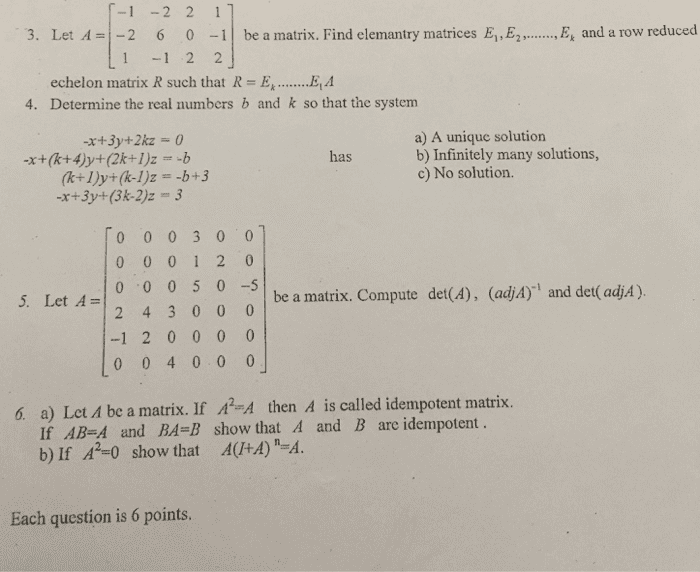
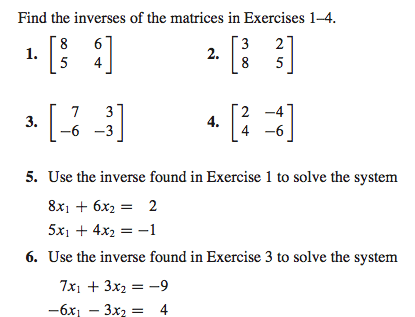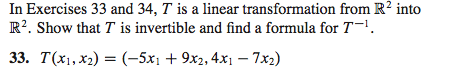MATH136 Lecture Notes - Coefficient Matrix, Elementary Matrix, Invertible Matrix

• School: University of Waterloo
• Department: Mathematics
• Course: MATH136
• Professor: Robert Sproule

Document Summary

Friday, march 21 lecture 30 : determinants and invertibility. Concepts: recognize that det(ab) = det(a)det(b), recognize that det(ca) = cndet(a), recognize that a matrix a is invertible iff det(a) 0, recognize that det(a-1) = 1 / det(a). 30. 1 theorem if a and b are two square matrices of same dimension then. Proof : we first see that if a is an elementary matrix e and b is any matrix, then det(ab) = det a det b: So the statement det(ab) = det a det b holds true if a is an elementary matrix. We prove det(ab) = det a det b by considering two cases. If a is not invertible then arref must have a row of zeroes. Now arref has a row of zeroes so det(arref) = 0 = det(a) as claimed. As previously shown, b must then be invertible. Since a is non-invertible ax = 0 has a non-zero solution, say u.