HomeClass Notes1,200,000CA670,000

MATH136 Lecture Notes - Lecture 18: Scalar Multiplication

78 views2 pages

ochresquirrel161

29 Apr 2014

School

University of Waterloo

Department

Course

Professor

For unlimited access to Class Notes, a Class+ subscription is required.

harlequinminnow989 and 36957 others unlocked

$MATH136 Full Course Notes$

MATH136 Full Course Notes

Document Summary

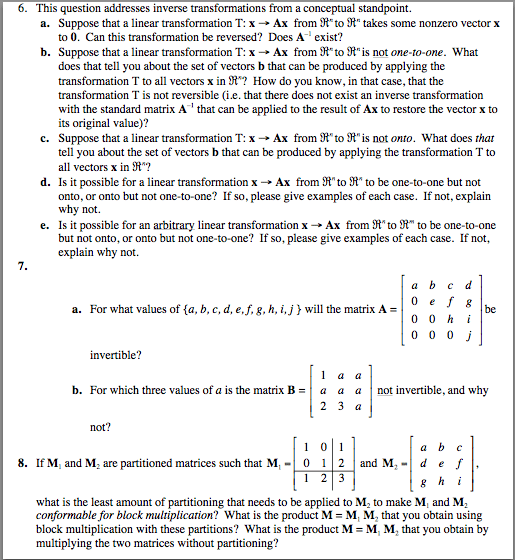
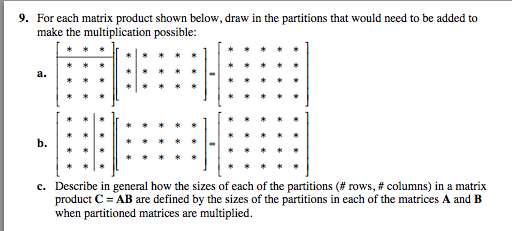
Friday, february 14 lecture 18 : operations on mappings. 18. 1 definition let t : n m and l : n m be two linear mapping. The mappings t and l are said to be equal mappings if and only if t(x) = l(x) for all x in their domain. Addition of mappings t + l is defined as (t + l)(x) = t(x) + l(x) Scalar multiplication of mapping t, for any scalar , is defined as ( t)(x) = t(x) be the matrices induced by t and l respectively. Then the matrix c = a + b is the unique matrix induced by the linear mapping t + l and the matrix a is the unique matrix induced by the linear mapping a t. 18. 2 theorem let t : n m and l : n m be two linear mapping. 18. 3 theorem let l denote the set of all linear mappings from n to m.

Related textbook solutions

Calculus

ISBN: 9781319050733

Single Variable Calculus: Early Transcendentals

4th Edition, 2018

ISBN: 9781337687805

CALCULUS:EARLY TRANSCENDENTALS

ISBN: 9781319050740

Related Documents

MATH136 Lecture Notes - Lecture 19: Spain, Matrix Addition, Scalar Multiplication

ochresquirrel161

MATH136 Lecture Notes - 32X, Solution Set, Augmented Matrix

amethystdolphin433

MATH136 Lecture Notes - Linear Combination, 2Wo

ochresquirrel161

Related Questions

linear algebra

Please help with all questions. Will rate ASAP.

This question addresses inverse transformations from a conceptual standpoint, a. Suppose that a linear transformation T: x rightarrow Ax from A to A takes some nonzero vector x to 0. Can this transformation be reversed? Does A exist? Suppose that a linear transformation T: x rightarrow Ax from is not one-to-one. What does that tell you about the set of vectors b that can be produced by applying the transformation T to all vectors x in A? How do you know, in that case, that the transformation T is not reversible (i.e. that there does not exist an inverse transformation with the standard matrix A-1 that can be applied to the result of Ax to restore the vector x to its original value)? Suppose that a linear transformation T: x rightarrow Ax from A to A is not onto. What does that tell you about the set of vectors b that can be produced by applying the transformation T to all vectors x in A? Is it possible for a linear transformation x rightarrow Ax from A to A to be one-to-one but not onto, or onto but not one-to-one? If so, please give examples of each case. If not, explain why not. Is it possible for an arbitrary linear transformation x rightarrow Ax from A to B to be one-to-one but not onto, or onto but not one-to-one? If so. please give examples of each case. If not, explain why not. For what values of {a. b. c, d. e.f. a. h. i.j } will the matrix A = invertible? what is the least amount of partitioning that needs to be applied to M; to make M1 and M2 conformable for block multiplication? What is the product M = M1, M2 that you obtain using block multiplication with these partitions? What is the product M = M1 M2. that you obtain by multiplying the two matrices without partitioning? For which three values of a is the matrix B = not invertible, and why If M1 and M2 arc partitioned matrices such that M1 For each matrix product shown below, draw in the partitions that would need to be added to make the multiplication possible: Describe in general how the sizes of each of the partitions(#rows, #columns) in a matrix product C = AB are defined by the sizes of the partitions in each of the matrices A and B when partitioned matrices are multiplied.