MATH136 Lecture 7: Week 3 (lecture 7-9) Lecture Notes

• School: University of Waterloo
• Department: Mathematics
• Course: MATH136
• Professor: Patrick Roh

Document Summary

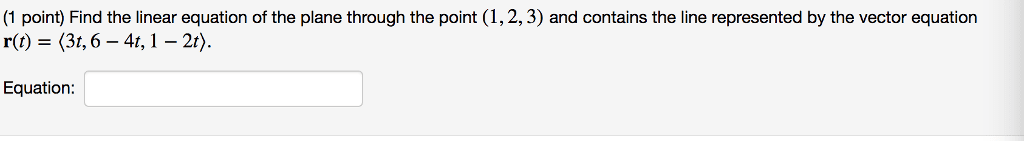
Lec 7 - shortest paths and system of linear equations. Find the shortest distance from the point to the plane. This plane does not pass through the origin. The vector does not go from the plane to . We need a vector from any point on the plane to . An equation of the form for constants is called a linear equation. The constants are the coefficients of the variables . A set of linear equations is called a system of linear equations. (note: a linear equation can be represented geometrically as a hyperplane. ) Eg is a linear equation. is not a linear equation. is a linear equation. (should be written as . ) (this is known as the standard form) In general, we will be looking at systems of linear equations in variables: Where is the coefficient of variable in the equation . Any vector is a solution of the system if the equations are satisfied when.