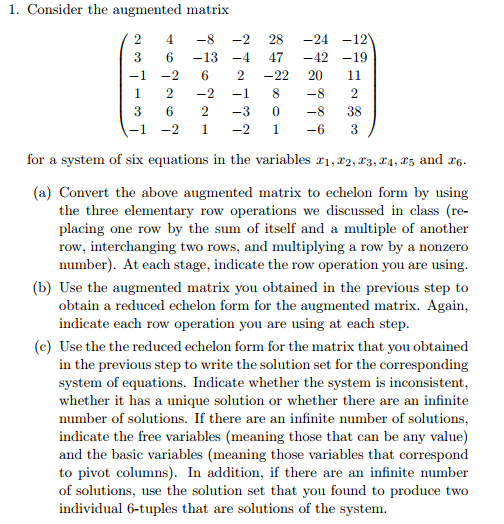
MATH 221 Lecture Notes - Augmented Matrix, Solution Set

19
MATH 221 Full Course Notes
Verified Note
19 documents
Document Summary
Encode into matrix system constants / co-efficients. Row operations to matrix system operations to linear system. Row operations: add a multiple of a row to another row, interchange two rows, multiplying a row by a nonzero scalar. Two matrices are row equivalent if one can be obtained from the other b a sequence of row operations. General strategy for solving a linear system: encode the system into an augmented matrix, use row operations to put the matrix into a simpler form, read off the solution from step 2. What do we mean by a simpler form in step 2. Each leading entry is in a column to the right of the leading entry of the row above: all of the entries in a column below the leading term are zero. ] row 3 6 (row 2) ~ [ ] (r3) / -5 not echelon form pivot but not leading entry of the final echelon form.