MATA30H3 Lecture Notes - Algebraic Solution, Algebraic Equation, Coefficient

39 views2 pages

ceruleanllama424

7 May 2013

School

Department

Course

Professor

For unlimited access to Class Notes, a Class+ subscription is required.

meghan78 and 39786 others unlocked

MATA30H3 Full Course Notes

Verified Note

27 documents

Document Summary

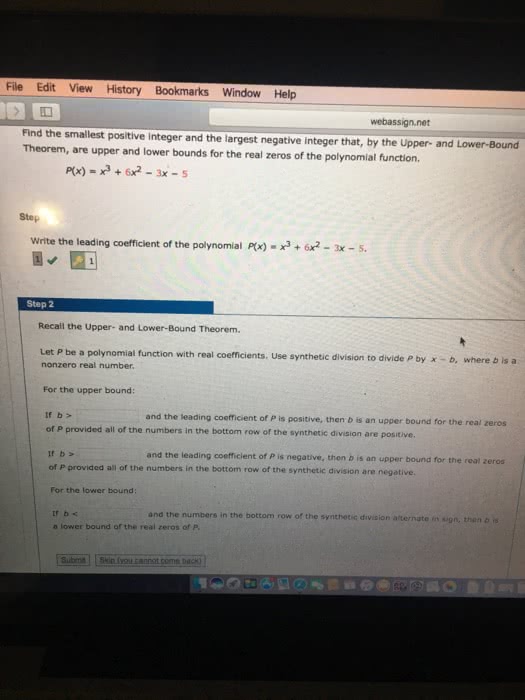
Long division can be used to divide a polynomial by a binomial. The result of the division of a polynomial function p(x) by a binomial of the form x b can be written as p(x) = (x-b)q(x) + r where q(x) is the quotient and r is the remainder. Division statement: divisor x quotient + remainder = dividend can be used to check the result of a division. For integer values of a and b, with a not equal 0, Factor theorem states that x b is a factor of a polynomial p(x) if and only if p(b) = 0. Similarly, if ax b is a factor of p(x) if and only if p(b/a) = 0. Integral zero theorem states that if x b is a factor of a polynomial function p(x) with leading coefficient 1 and remaining coefficients that are integers, then b is a factor of the constant term p(x).

Related Questions

A polynomial P(x) and a divisor d(x) are given. Use long divisonto find the quotient Q(x) and the remainder R(x). Express P(x) inthe form P(x)= d(x) times Q(x)+R(x)

P(x)=x³-512

d(x)=x+8

2. For the function F(x), find the maximum number of real zeros,the maximum number of x-intercepts, and the maximum number ofturning points that the function can have

F(x)=x⁵-x³+7

3. Use synthetic divison to find P(k) for the given value of kand the given function P.

k=3;P(x)=x³-2x²+8

4. Use synthetic division to find the quotient and theremainder.

(x³-3x²-6) divided by (x+2)

5. Suppose a polynomial function of degree 4 with rationalcoefficients has the given numbers as zeros. Find the otherzeros.

1+5i,5+square root of 5