MAT224H1 Lecture : Rank Nullity Theorem, coordinates, matricies of transformation
Document Summary
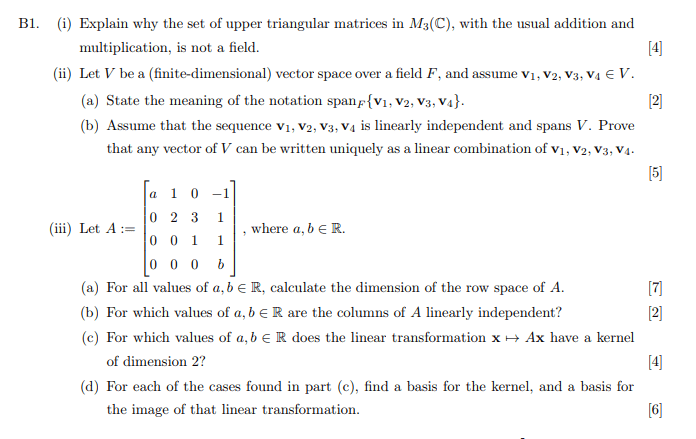
A very simple example of kernel and image (with picture): Let f : r2 r2 be given by f ((x onto the x-axis. The kernel is by de nition the set of all vectors (x that their image under f is the zero vector, i. e. {(x ker f = h(0: , i. e. the orthogonal projection, such. Thus y)) = (x: | (x, = (0. The image of f is by de nition the set of all images of vectors (x: under f : imf = {f (x, | (x, r2} = {(x, |x r} = h(1. It turns out that the rank and the nullity of a transformation are always related by a very simple formula, called rank-nullity theorem. Let f : v w be a linear transforma- tion of k-vector spaces. Then dim ker f + dim imf = dim v. The subspace ker f is nite-dimensional, since it is a subspace of a.