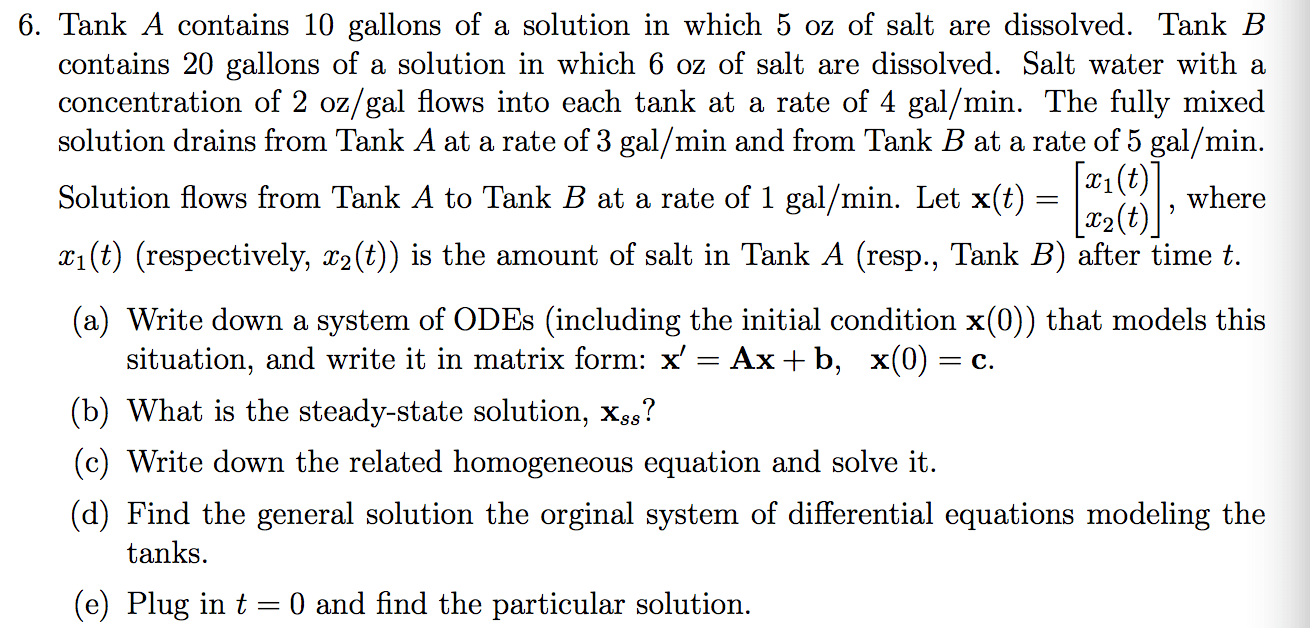
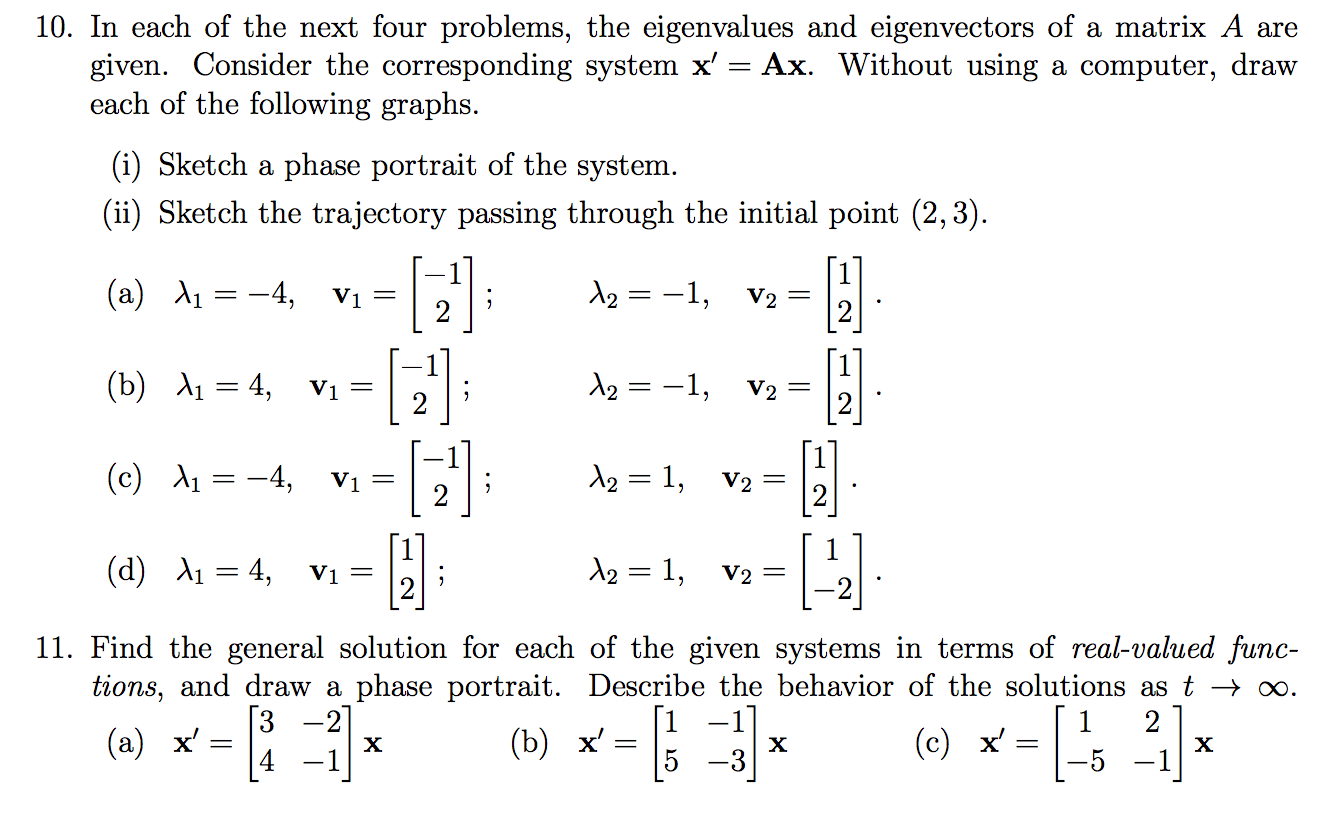
18.03 Lecture Notes - Lecture 28: Diagonalizable Matrix, Matrix Exponential, Identity Matrix
Document Summary
Express solutions to homogeneous systems of odes in terms of a fundamental matrix. Decompose a non-diagonalizable matrix into components b + c = a such that bc = cb to nd. Find a fundamental matrix of a system with complex eigenvalues. Consider a homogeneous linear (constant coef cient) system of n odes x" = ax. We know that the set of solutions is an n-dimensional vector space. Let x , , x be a basis of solutions. Write x , , x as column vectors side by side to form a matrix. Any such x(t) is called a fundamental matrix for x" = ax. The general solution to x" = ax is. Conclusion: if x(t) is a fundamental matrix, then the general solution is x(t)c, where c ranges over constant vectors has eigenvalue 2 with eigenvector and eigenvalue 3 with eigenvector so one fundamental matrix is.