MATH 251 Lecture Notes - Fourier Series, Periodic Function
Document Summary
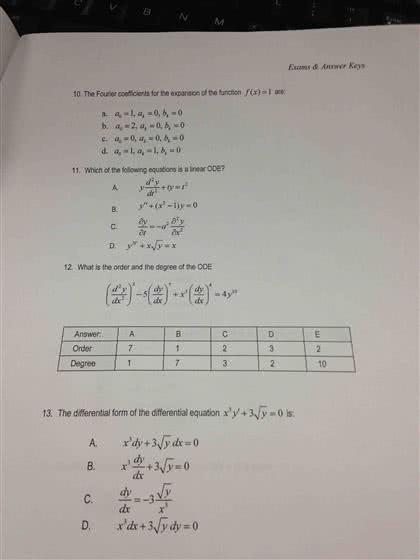
Suppose f is a piecewise continuous periodic function of period 2l, then f has a fourier series representation xf a. Where the coefficients a"s and b"s are given by the euler-fourier formulas: a m. L dx m = 0, 1, 2, 3, b n. Theorem: suppose f and f are piecewise continuous on the interval. L x l. further, suppose that f is defined elsewhere so that it is periodic with period 2l. Then f has a fourier series as stated above whose coefficients are given by the euler-fourier formulas. The fourier series converge to f (x) at all points where f is continuous, and to lim x c xf lim)( c x xf. 2/)( at every point c where f is discontinuous. If f is an even periodic function of period 2l, then its fourier series contains only cosine (include, possibly, the constant term) terms. That is, its fourier series is of the form xf a.