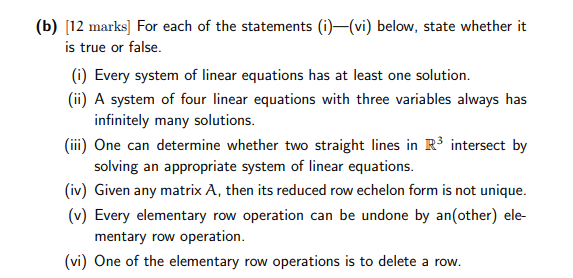
1. Consider the augmented matrix: (linear algebra)
a) Convert the matrix to echelon form
b) Obtain the reduced echelon form from the previous step
c) write a solution set for the corresponding system of equations
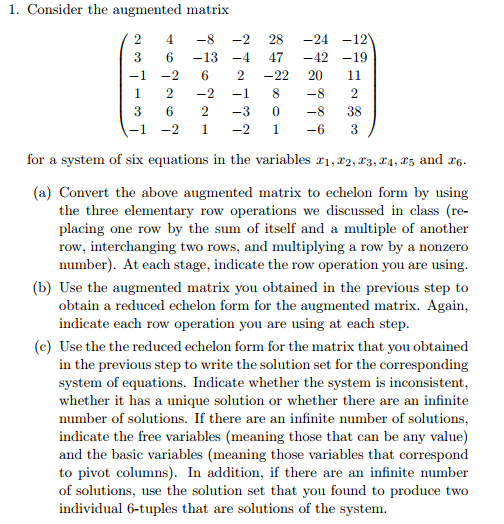
1. Consider the augmented matrix 2 4-8-2 28-24 -12 3 6 -13 47-42 -19 -1 -2 62-22 20 11 2 -3 0 -838 2 -63 1 2-2 -1 8-8 3 6 2 for a system of six equations in the variables r1, 2, r3, r4, r5 and re (a) Convert the above augmented matrix to echelon form by using the three elementary row operations we discussed in class (re- placing one row by the sum of itself and a multiple of another row, interchanging two rows, and multiplying a row by a nonzero number). At each stage, indicate the row operation you are using (b) Use the augmented matrix you obtained in the previous step to obtain a reduced echelon form for the augmented matrix. Again, peration you are using at each step. (c) Use the the reduced echelon form for the matrix that you obtained in the previous step to write the solution set for the corresponding system of equations. Indicate whether the system is inconsistent whether it has a unique solution or whether there are an infinite number of solutions. If there are an infinite number of solutions, an be any value) and the basic variables (meaning those variables that correspond to pivot columns). In addition, if there are an infinite number of solutions, use the solution set that you found to produce two indicate the free variables (meaning those that c individual 6-tuples that are solutions of the system.