MAT135H1 Final: 3.2 Max and Min Values on an Interval

144
MAT135H1 Full Course Notes
Verified Note
144 documents
Document Summary
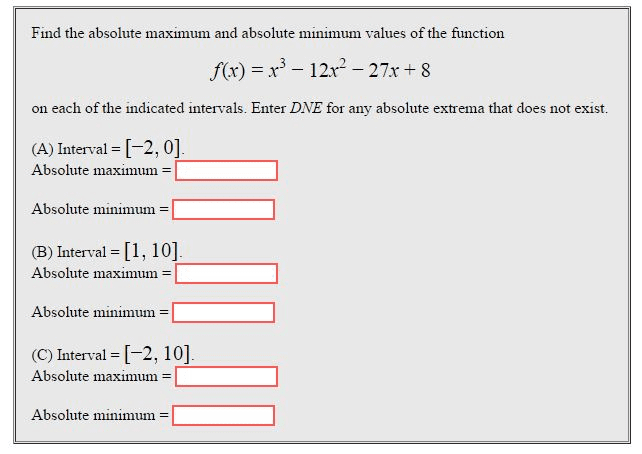
For f x on an interval [a, b: find the derivative, find all points in the interval [a, b] where, evaluate, compare the value is step 3: x f f. 0 x = f x at the endpoints of the function [a, b], and points where f. 0 x = the largest value is the max value of f x on the interval the smallest value is the min value of f x on the interval. Example 1: find the extreme values of the function f x. Step 1: find the derivative, f x f x. Step 2: find all points in the interval [a, b] where f. Step 3: evaluate at x o at x 3. 3 tx. az both are withininternal valid f x at the endpoints of the function [a, b], and points where f. Step 4: compare the value is step 3. 1311 max absolute min is at x 5 absolute max is at x 3.