MATH 111 Midterm: MATH 111 NJIT M111 F17 CE3 Solutions

1
Math 111 Final Exam
December 15, 2017
Time: 2 hours and 30 minutes
Instructions: Show all work for full credit.
No outside materials or calculators allowed.
Extra Space: Use the backs of each sheet
for extra space. Clearly label when doing so.
Name:
ID #:
Instructor/Section:
“I pledge by my honor that I have abided by the
NJIT Academic Integrity Code.”
(Signature)
1.
Consider the function
2
( ) 1.y fx x= = −
(a) Sketch f, noting that
2
1x−
is negative for
11x−< <
. (Hint: Plot
2
1.yx= −
) (3 pts.)
(b) Show that the function is continuous everywhere. (2 pts.)
(c) Show
()fx
′
is not continuous at x =1 by showing
11
lim ( ) lim ( ).
xx
fx fx
→− →+
′′
≠
(5 pts.)
Solution 1.
(a) The sketch is
(b) As the function is equal to
21x−
for
1x>
and
2
1x−
for
1x<
, which are continuous, it is only
necessary to prove continuity at
1.x= ±
Since
2
10x−→
as
1 ,1x−+
→−
and
2
10x−→
as
1 ,1 ,x+−
→−
we see
11
lim ( ) lim ( ) 0 ( 1) (1),
xx
fx fx f f
→− →
= == −=
so the continuity follows.
(c) As
() 2fx x
′=
for
1x>
and
() 2fx x
′= −
for
0 1,x<<
we compute that
11
11
lim ( ) lim ( 2 ) 2 2 lim ( ) lim (2 ).
xx
xx
fx x fx x
−−
→+ →+
→→
′′
= − =−≠ = =
Problem Value Score
1 10 pts.
2 10 pts.
3 15 pts.
4 10 pts.
5 15 pts.
6 10 pts.
7 15 pts.
8 15 pts.
TOTAL 100

2
2.
Let
()y yx=
be implicitly defined by
1 sin( ).
x
y e xy= +
(a) Compute the derivative dy/dx. (5 pts.)
(b) Find the tangent line to the curve at
( ,1).
π
. (5 pts.)
Solution 2.
(a) Assuming
()y yx=
is differentiable, we compute by implicit differentiation that
[ ]
[ ]
sin( ) cos( ) 1 cos( ) sin( ) cos( )
sin( ) cos( ) .
1 cos( )
xx x x
x
x
dy dy dy
e xy e xy y x xe xy e xy y xy
dx dx dx
e xy y xy
dy
dx xe xy
= + + ⇒− = + ⇒
+
=−
(b) At
( ,1)
π
it follows from (a) that
[ ]
sin (1) cos
() .
1 cos 1
e
dy e
dx e e
ππ
ππ
ππ
πππ π
+−
= =
−+
Hence, the tangent line at
( ,1)
π
is
()()
1.
11
ex e x
yee
ππ
ππ
ππ
ππ
−− −
−= =
++
3
3.
(a) A rectangular yard is to be completely enclosed by fencing and then divided into three
enclosures of equal area by fences parallel to and of the same length as one side of the
yard. If 400 ft. of fencing is available, what dimensions maximize the enclosed area?
Verify that your answer is the largest possible area. (8 pts)
(b) Find a positive number for which the sum of its reciprocal and four times its square is
the smallest possible. Verify that your answer is the smallest possible sum. (7 pts.)
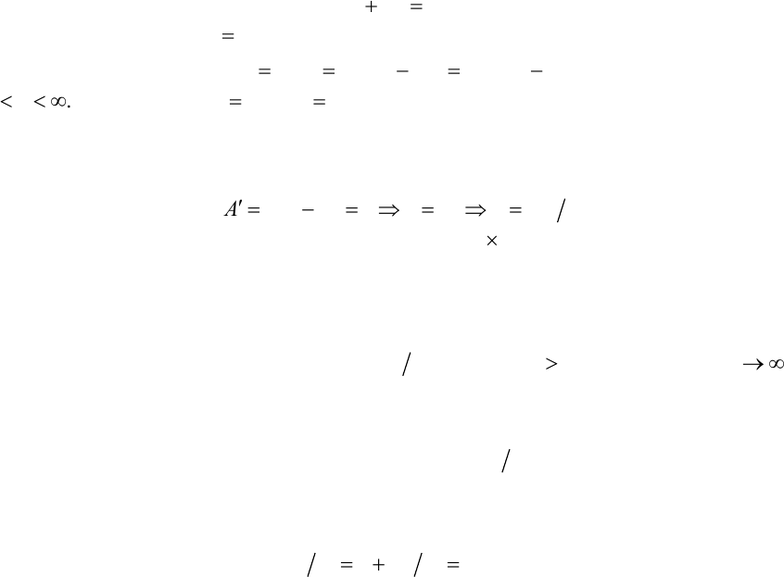
Solution 3.
(a) Let x be the length of each of the four parallel fences for the three equal enclosures and 6y be the
lengths of the remaining fencing. Then, we are given that
4 6 400.xy+=
We have to maximize the area
(3 )Axy=
subject to the above constraint, so we maximize
( ) (200 2 ) 2 (100 )A Ax x x x x= = −= −
for
0.x< <∞
Noting that
(0) (100) 0AA= =
and that A is negative for x > 100, it follows that we can
confine our attention to the closed interval [0,100] on which A has a maximum since it is continuous on
the whole real line. The maximum must occur at an interior point of the interval which must be a critical
point, so we compute that
200 4 0 50 100 3,A xx y
′= − =⇒= ⇒=
so it follows that the maximum area of the rectangular yard is
50 100×
ft.2 . It is a local maximum by the
first derivative or second derivative test (the first derivative changes sign from positive to negative across
x = 50 and the second derivative is -4, so the graph is concave down on the interval [0,100]). It is the only
critical point of A(x), so it must yield a global maximum.
(b) Here we need to find the minimizer of
2
( ) (1 ) 4Sx x x= +
for
0.x>
Noting that
()Sx→∞
as
0, ,x+
→∞
we conclude that S must have a minimum on a bounded closed positive interval. Moreover,
the function is infinitely differentiable for x > 0, so the minimum must occur at a critical point. Now
28 0 1 2,Sx x x
−
′=− + =⇒=
and
3
() 2 8Sx x
−
′′ = +
, which is concave up for all x > 0. Consequently, the absolute (global)
minimum is
(1 2) 2 4(1 4) 3,S=+=
which is attained at x = 1/2.
