MATH 32A Midterm: MATH32A Midterm 2 2010 Spring
Document Summary
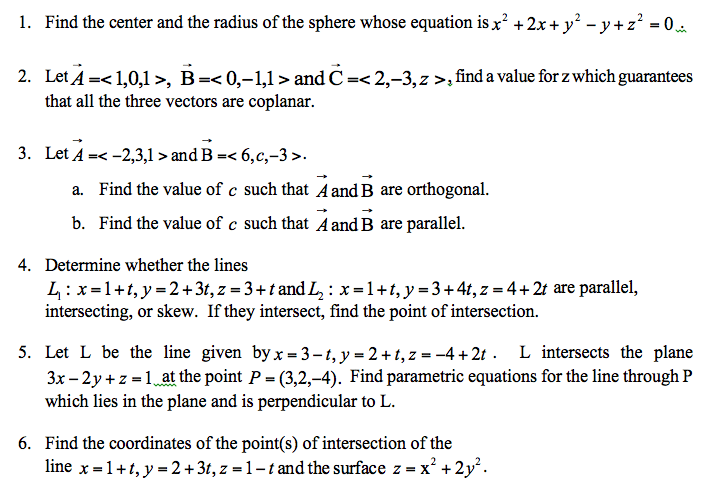
Try to answer the following questions without the use of book, notes or calculator. Time yourself and try to nish the test in less than 50 minutes: a particle moves through three dimensional space with velocity v(t) = (cid:104)sec2 t, 2 sec t tan t, tan2 t(cid:105). At time t = 0 the particle is at (cid:104)0, 1, 2(cid:105), nd the position function of the particle for. /4 t /4: find the cumulative length function s(t) (starting from a = 1) of the parametric curve (cid:104)ln t, 2 t2(cid:105): sketch in the xy-plane the domain of f (x, y) =