CHEM10004 Chapter Notes -Manganese Nodule, Coordination Complex, Paramagnetism
Document Summary
Get access


Related textbook solutions
Chemistry: Structure and Properties
Basic Chemistry
Principles of Chemistry Molecular Approach
Principles of Chemistry Molecular Approach
Chemistry: Structure and Properties
Chemistry: A Molecular Approach
Chemistry: A Molecular Approach
Principles of Chemistry: A Molecular Approach
Chemistry: The Central Science
Related Documents
Related Questions
MC15/20 AnimationâAnalysis of Copper-Zinc Voltaic Cell
Some oxidation-reduction reactions are spontaneous, and the energy released by them can be used for electrical work. This principle is used in the working of a voltaic cell. Voltaic cells, or electrochemical cells, make use of the electrons that are transferred from the species that releases electrons and undergoes oxidation to the species that accepts electrons and undergoes reduction. If it is possible by any means to separate the oxidation reaction and the reduction reaction and then connect them externally so that the electrons flow from one compartment to the other, you can construct an electrochemical cell.
Watch the video that describes the cell reaction and the cell components of a voltaic cell.
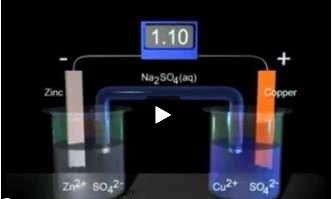
In a copper-zinc voltaic cell, one half-cell consists of a Zn electrode inserted in a solution of zinc sulfate and the other half-cell consists of a Cu electrode inserted in a copper sulfate solution. These two half-cells are separated by a salt bridge.
At the zinc electrode (anode), Zn metal undergoes oxidation by losing two electrons and enters the solution as Zn2+ ions. The oxidation half-cell reaction that takes place at the anode is
Zn(s)âZn2+(aq)+2eâ
The Cu ions undergo reduction by accepting two electrons from the copper electrode (cathode) and depositing on the electrode as Cu(s). The reduction half-cell reaction that takes place at the cathode is
Cu2+(aq)+2eââCu(s)
The electrons lost by the Zn metal are gained by the Cu ion. The transfer of electrons between Zn metal and Cu ions is made possible by connecting the wire between the Zn electrode and the Cu electrode. Thus, in the voltaic cell, the electrons flow through an external circuit from the anode to the cathode. For a voltaic cell to work, the solution in the two half-cells must remain electrically neutral. This can happen only if the flow of ions is countered with the flow of electrons. The flow of ions is made possible with the use of a salt bridge. A salt bridge is a solution of some other metal that has common ions. If a copper-zinc voltaic cell utilizes ZnSO4 and CuSO4solution, you will use a saturated Na2SO4 solution in the salt bridge. Thus, the salt bridge will help the migration of ions across the two compartments, or two half-cells.
Part A
Label the diagram according to the components and processes of a voltaic cell.
Drag the appropriate labels to their respective targets.
Hints
| Zinc Cathode Flow of cations Flow of electrons Flow of anions Copper Cathode Zinc Anode Copper Anode Reduction half-cell Oxidation half-cell Help Reset |
SubmitMy AnswersGive Up
Correct
The cell reaction for the voltaic cell is
Zn(s)+Cu2+(aq)âZn2+(aq)+Cu(s)
Here, Zn undergoes oxidation by losing 2eâ, and the Cu2+ ions accept 2eâ to form metallic Cu, which is deposited at the copper electrode. At the zinc electrode, oxidation occurs, and hence it is known as the anode. Reduction occurs at the copper electrode, and hence it is called the cathode.
The electrochemical cell notation for this cell is Zn(s)|Zn2+(aq)||Cu2+(aq)|Cu(s).
Construction of a voltaic cell
Consider a chromium-silver voltaic cell that is constructed such that one half-cell consists of the chromium, Cr, electrode immersed in a Cr(NO3)3 solution, and the other half-cell consists of the silver, Ag, electrode immersed in a AgNO3 solution. The two electrodes are connected by a copper wire. The Cr electrode acts as the anode, and the Ag electrode acts as the cathode. To maintain electric neutrality, you add a KNO3 salt bridge separating the two half-cells. Use this information to solve Parts B, C, and D.
Part B
The half-cell is a chamber in the voltaic cell where one half-cell is the site of the oxidation reaction and the other half-cell is the site of the reduction reaction.
Type the half-cell reaction that takes place at the anode for the chromium-silver voltaic cell. Indicate the physical states using the abbreviation (s), (l), or (g) for solid, liquid, or gas, respectively. Use (aq) for an aqueous solution. Do not forget to add electrons in your reaction.
Express your answer as a chemical equation.
Hints
| | ||
SubmitMy AnswersGive Up
Part C
The half-cell is a chamber in the voltaic cell where one half-cell is the site of an oxidation reaction and the other half-cell is the site of a reduction reaction.
Type the half-cell reaction that takes place at the cathode for the chromium-silver voltaic cell. Indicate physical states using the abbreviation (s), (l), or (g) for solid, liquid, or gas, respectively. Use (aq) for an aqueous solution. Do not forget to add electrons in your reaction.
Express your answer as a chemical equation.
Hints
| | ||
