I posted the chart and questions. I will still write them if they are not clear.
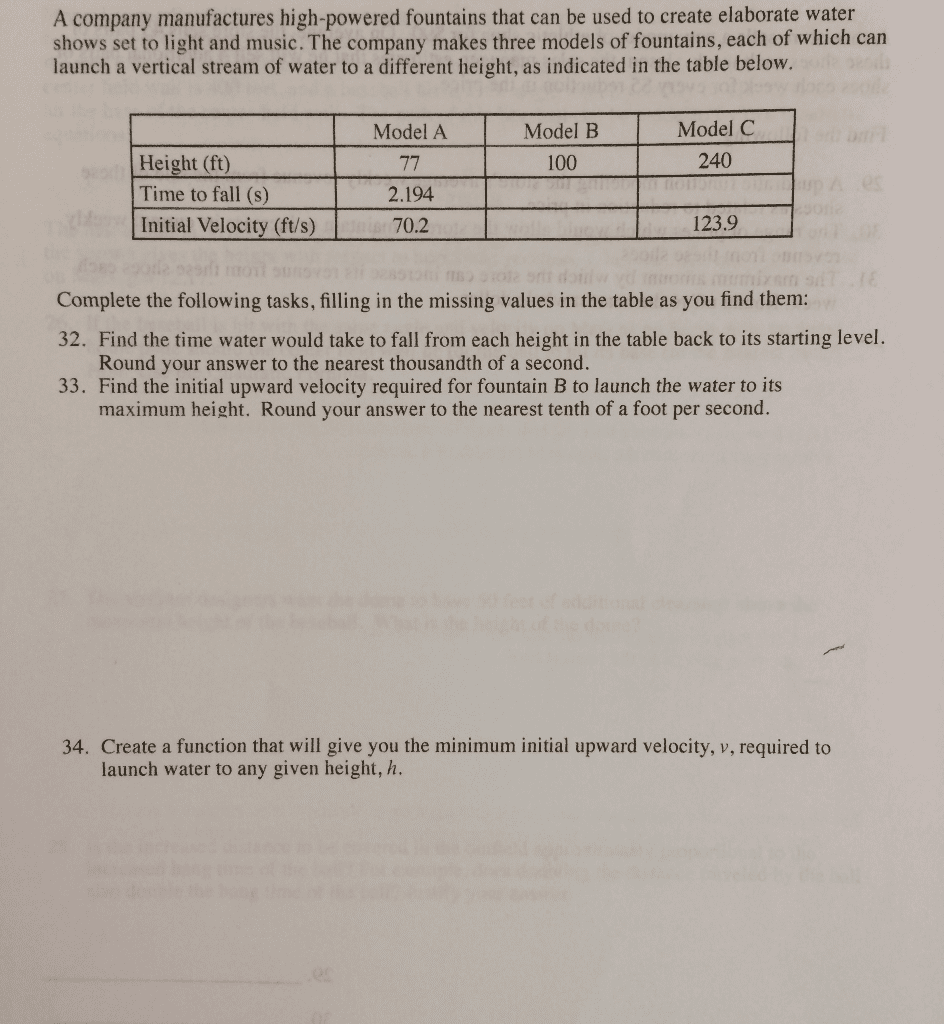
A company manufactures high powered fountains that can be used to create elaborate water shows set to light and music. The company makes three models of fountains, each of which can launch a vertical stream of water to a different height, as incdicated in the table above ( see picture).
Complete the following tasks, filling in the misssing values in the table back to its starting level.
32) Find the time water would take to fall from each height in the table back to its starting level. Round your answer to the nearest thousandth of a second.
33) Find the initial upward velocity required for Fountain B to launch the water to its maxiumum height. Round your answer to the nearest tenth of a foot per second.
34) Create a function that will give you the minimum initial upward velocity, v , required to launch water to any given height, h.
You can see the image here too: http://imgur.com/a/n0n9D
Also there is another question i am stuck on: Here it is
Consider the properties of complex numbers.
9) Which of the following is true about the complex number a + bi
A) a and b are both real
B) a is real and b is imaginary
C) a is imaginary and b is real
D) a and b are both imaginary
E) all of the above
My text book says a complex number written in standard form is a number a + bi where a and b are real numbers. The number a is the real part of the complex number, and the number bi is the imaginary part.
So i know that A is correct but i am confused for option B, because isn't bi the imaginary part?
Thanks for whoever helps, it means alot.