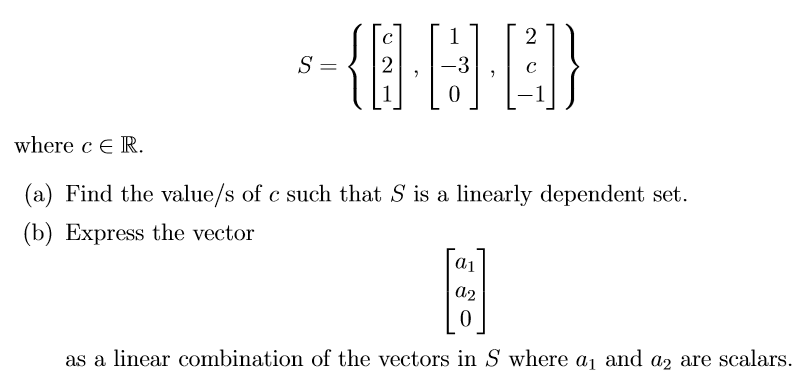
Please Show Work.
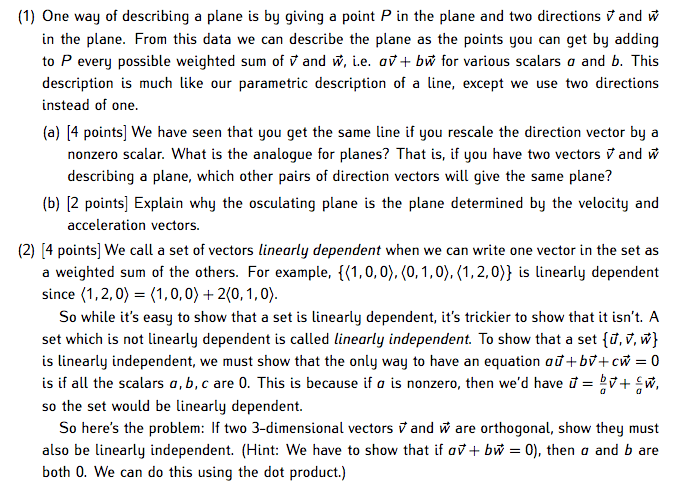
One way of describing a plane is by giving a point P in the plane and two directions v and w in the plane. From this data we can describe the plane as the points you can get by adding to P every possible weighted sum of v and w, i.e. av + bw for various scalars a and b. This description is much like our parametric description of a line, except we use two directions instead of one. [4 points] We have seen that you get the same line if you rescale the direction vector by a nonzero scalar. What is the analogue for planes? That is, if you have two vectors v and w describing a plane, which other pairs of direction vectors will give the same plane? [2 points] Explain why the osculating plane is the plane determined by the velocity and acceleration vectors. [4 points] We call a set of vectors linearly dependent when we can write one vector in the set as a weighted sum of the others. For example, {(1,0,0), (0,1,0), (1,2,0)} is linearly dependent since (1,2,0) = (1,0,0) + 2(0,1,0). So while it's easy to show that a set is linearly dependent, it's trickier to show that it isn't. A set which is not linearly dependent is called linearly independent. To show that a set {u, v, w} is linearly independent, we must show that the only way to have an equation au + bv + cw = 0 is if all the scalars a,b,c are 0. This is because if a is nonzero, then we'd have u = b/a v + c/a w, so the set would be linearly dependent. So here's the problem: If two 3-dimensional vectors v and w are orthogonal, show they must also be linearly independent. (Hint: We have to show that if av + bw = 0), then a and b are both 0. We can do this using the dot product.)
Show transcribed image text One way of describing a plane is by giving a point P in the plane and two directions v and w in the plane. From this data we can describe the plane as the points you can get by adding to P every possible weighted sum of v and w, i.e. av + bw for various scalars a and b. This description is much like our parametric description of a line, except we use two directions instead of one. [4 points] We have seen that you get the same line if you rescale the direction vector by a nonzero scalar. What is the analogue for planes? That is, if you have two vectors v and w describing a plane, which other pairs of direction vectors will give the same plane? [2 points] Explain why the osculating plane is the plane determined by the velocity and acceleration vectors. [4 points] We call a set of vectors linearly dependent when we can write one vector in the set as a weighted sum of the others. For example, {(1,0,0), (0,1,0), (1,2,0)} is linearly dependent since (1,2,0) = (1,0,0) + 2(0,1,0). So while it's easy to show that a set is linearly dependent, it's trickier to show that it isn't. A set which is not linearly dependent is called linearly independent. To show that a set {u, v, w} is linearly independent, we must show that the only way to have an equation au + bv + cw = 0 is if all the scalars a,b,c are 0. This is because if a is nonzero, then we'd have u = b/a v + c/a w, so the set would be linearly dependent. So here's the problem: If two 3-dimensional vectors v and w are orthogonal, show they must also be linearly independent. (Hint: We have to show that if av + bw = 0), then a and b are both 0. We can do this using the dot product.)