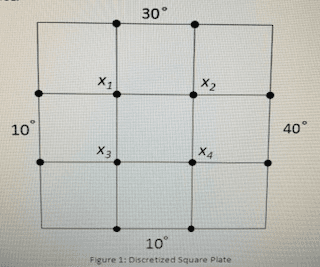
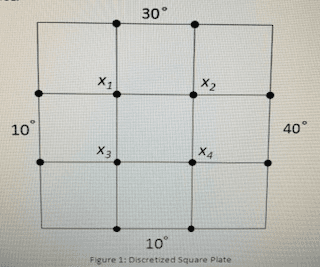
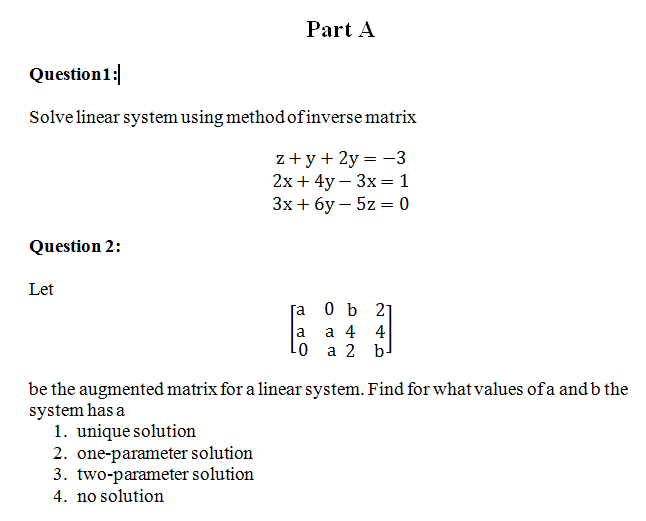
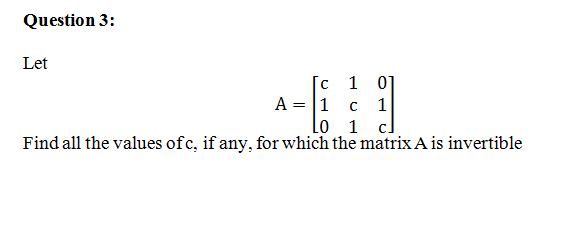MATH136 Lecture Notes - Cross Product, Vandermonde Matrix, Parallelepiped

34
MATH136 Full Course Notes
Verified Note
34 documents
Document Summary
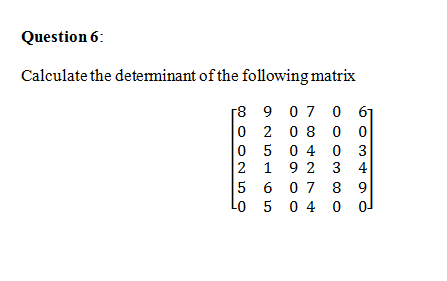
Monday, march 24 lecture 31 : determinant applications. Cramer"s rule for solving systems of linear equations using. If a is an invertible n n matrix and b is a vector in n, then ai(b) denotes the matrix obtained by replacing the ith column of a by b. The unique solution x of the system ax = b is given by. Expanding along row 1 we get det a = 6. 31. 2 definition let a be an n n matrix. The adjoint of a, adj(a), (also called the adjugate of a) is the transpose of the matrix whose entries are the cofactors cij of a. 31. 2. 1 example find the (1, 2)th entry of the adjoint, adj(a), of the matrix. Since d = adj(a) = [ cij ]t then d12 = c21. Once computed the complete matrix d = adj(a) is. 31. 3 theorem adjoint method of finding the inverse of a. (also called the cofactor method).