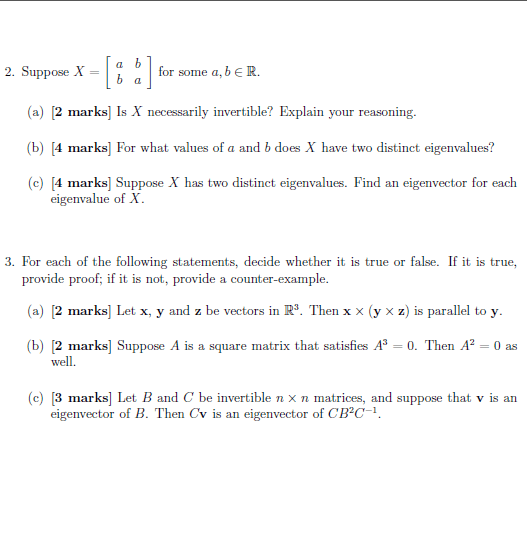
MATH136 Lecture Notes - Free Variables And Bound Variables, Eigenvalues And Eigenvectors, Main Diagonal

34
MATH136 Full Course Notes
Verified Note
34 documents
Document Summary
April 2 lecture 35 : diagonalizable matrices. Concepts: define diagonalizable n n matrix a, recognize that a matrix a is diagonalizable if the matrix a has n distinct, diagonalize simple 2 by 2 or 3 by 3 matrices. eigenvalues. 35. 1 theorem suppose a is an n n matrix with n distinct eigenvalues. Let x be the n n matrix whose columns are the n eigenvectors which are respectively associated to the n distinct eigenvalues of a matrix a. Given : suppose {v1, v2, , vn} are n distinct eigenvectors respectively associated to n distinct eigenvalues { 1, 2, , n}. Required to show : that a = [v1 v2 vn ] is invertible. Then one vector, say v1, is a linear combination of the others {v2, , vn}. If this set {v2, , vn} is not linearly independent we can reduce it to a smaller linearly independent set, say {v2, , vm}.