MATA31H3 Lecture 5: week 5

44 views11 pages

brownturtle157

23 Oct 2017

School

Department

Course

Professor

For unlimited access to Class Notes, a Class+ subscription is required.

Document Summary

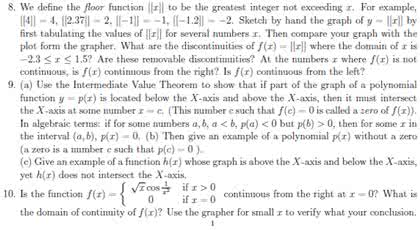
5. 1 continuity at a point and on an interval. Intuitively we thought of continuous functions as of functions with graphs we can sketch. Without picking up our pencil in this section we"ll develop a formal definition of continuity using limits. , we say that f is continuous at c if (c p, c + p) f (x) = f (c) lim x c. Graph of continuous function f is continuous at every number in an interval (has no breaks in this interval) The above implies: is defined (c is in the domain of f(x)) f (c) lim x c lim x c f (x) exists f (x) = f (c) f (x) Types of discontinuities is discontinuous at c if one of the (1), (2), or (3) fails. If function f defined on open interval (c p, c + p) , we say that f is continuous at c if for each.

Related Questions

Can you please help me with number 5?

Show transcribed image text

bluebee563

if possible, please show steps. thanks

1. (18 pts) The graph of a function f, defined on (-0, +o), is given in the figure below. - - y = f(x) - - - - - - N - - 4 A -2-1 144 -1 - - - - - - - -- - - -- Use the graph of f to answer questions (a)-(f) below. (a) Determine the value or write "does not exist". i. f(-3) = -2 ii. f(0) = 1 (b) Determine the limit or write "does not exist" (DNE). Write "does not exist" only if a limit does not exist and is not too or -0. i. lim f(x) = 0 iv. lim f(x) = +00 X- 3 x2 ii. lim f(x) = -4 v. lim f(x) = -0 X- 0- x -00 iii. lim f(x) = DNE vi. lim f(x) = -2 x0 x++00 (C) MULTIPLE CHOICE! Circle ONLY one answer! Find a number a for which the following statement is true (the function f is shown above): STATEMENT: The function f is continuous at a, and lim f(x) = 0. i. a=-3 iii. a=0 V. a=3 ii. a = -2 iv. a = 2 vi. Such a number does not exist. (d) Determine the largest intervals of continuity of f. Use interval notation to write your answer. (-0, -3), (-3,0), (0, 2), (2, +00) (e) Write the equation(s) of all vertical asymptotes of f. Write "none" if appropriate. x= 2 (f) Write the equation(s) of all horizontal asymptotes of f. Write "none" if appropriate. y = -2

MATA31H3 Lecture 5: week 5

Document Summary

Get access

Related Documents

MATA31H3 Lecture Notes - Lecture 7: Triangle Inequality, Computer-Aided Technologies

MATA31H3 Lecture Notes - Lecture 13: Inflection Point, Maxima And Minima, Even And Odd Functions

MATA31H3 Lecture Notes - Lecture 10: Tuu Languages, Quality Function Deployment, Mean Value Theorem

Related Questions