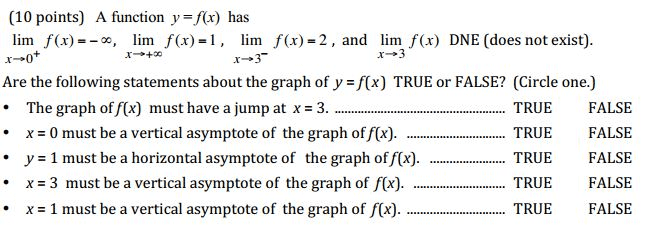MAT237Y1 Lecture : 4.3 exponential.pdf
16 views4 pages
Document Summary
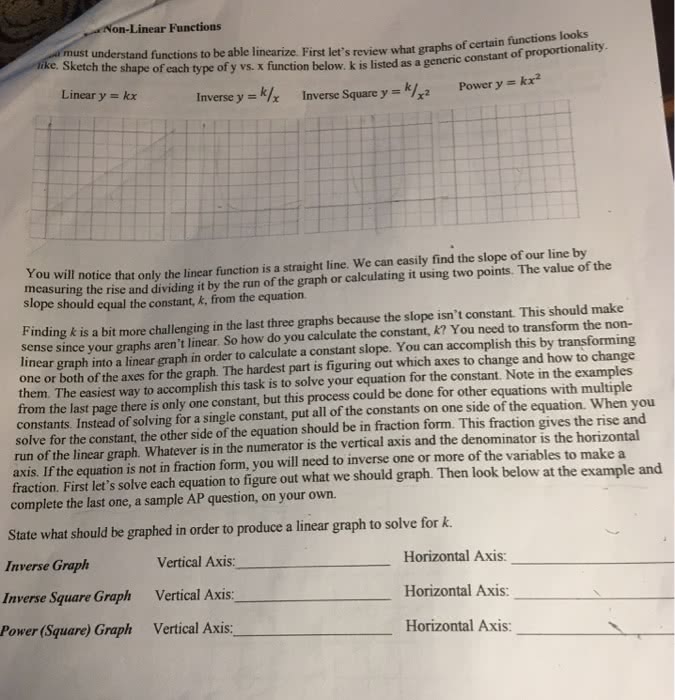
4. 3 - derivatives of exponential and inverse trig functions. Remember, in order for the inverse of a function to exist, the function must be one-to-one. Ie, it must pass the horizontal line test. Think about how the inverse is a re ection over the line y = x. In order for f 1 to be di erentiable, we don"t want it to have any vertical tangents. Okay, so basically, in order for f 1 to exist and be di erentiable, f needs to be one-to-one and have no horizontal tangents. So now we must ask ourselves, what is the derivative of the inverse of a function? . Or, if you want to think of it this way, dy dx. Find the derivative of the inverse. x = y y + 1 x(y + 1) = y xy + x = y x = y xy x = y(1 x) y = x.
Get access
Grade+20% off
$8 USD/m$10 USD/m
Billed $96 USD annually

Homework Help
Study Guides
Textbook Solutions
Class Notes
Textbook Notes
Booster Class
40 Verified Answers
Class+
$8 USD/m
Billed $96 USD annually

Homework Help
Study Guides
Textbook Solutions
Class Notes
Textbook Notes
Booster Class
30 Verified Answers