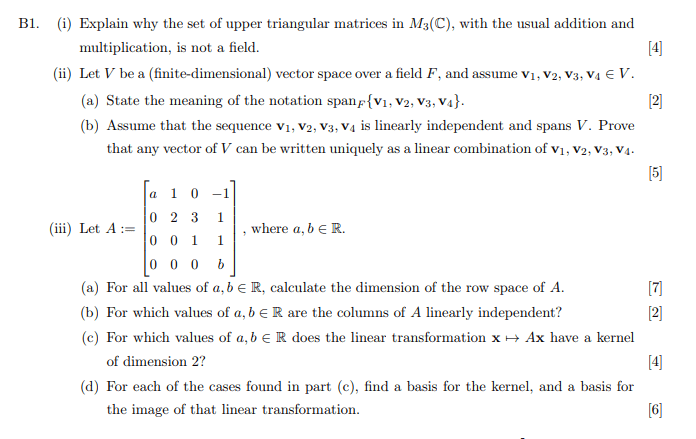
B1. (i) Explain why the set of upper triangular matrices in M3(C), with the usual addition and multiplication, is not a field. (ii) Let V be a (finite-dimensional) vector space over a field F, and assume vi, V2, v3,V4EV. (a) State the meaning of the notation spanf{vi, V2, v3, vi) (b) Assume that the sequence V1,V2,V3.V4 ?s linearly independent and spans V. Prove 2 that any vector of V can be written uniquely as a linear combination of v, v2, V3, V4 a 1 0 -1 (iüi) Let A0 2 3 1 000b where a, beR (a) For all values of a, bER, calculate the dimension of the row space of A (b) For which values of a, b R are the columns of A linearly independent? (c) For which values of a, b E R does the linear transformation x +> Ax have a kernel of dimension 2? (d) For each of the cases found in part (c), find a basis for the kernel, and a basis for the image of that linear transformation. 6

