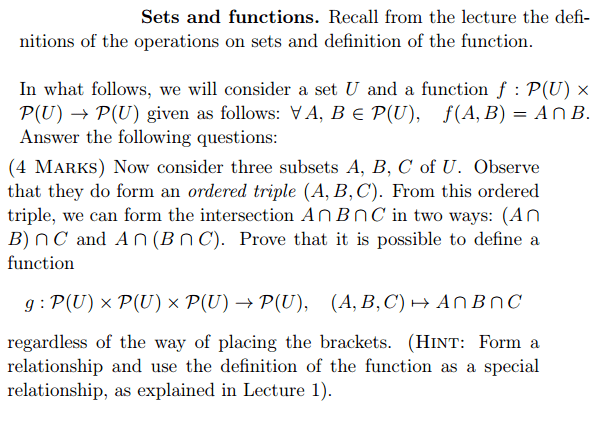
Sets and function. Recall from the lecture the definitions of the operations on sets and definition of the function. In what follows, we will consider a set U and a function f: P(U) times P(U) rightarrow P(U) given as follows: forall A, B element P(U), f(A, B) = A intersection B. Answer the following questions Now consider three subsets A, B, C of U. Observe that they do form an ordered triple (A, B, C). From this ordered triple, we can form the intersection A intersection B intersection C in two ways: (A intersection B) intersection C and A intersection (B intersection C) Prove that it is possible to define a function g P(U) times P(U) times P(U) rightarrow P(U), (A, B, C) rightarrow A intersection B intersection c regardless of the way of placing the brackets.
Show transcribed image text Sets and function. Recall from the lecture the definitions of the operations on sets and definition of the function. In what follows, we will consider a set U and a function f: P(U) times P(U) rightarrow P(U) given as follows: forall A, B element P(U), f(A, B) = A intersection B. Answer the following questions Now consider three subsets A, B, C of U. Observe that they do form an ordered triple (A, B, C). From this ordered triple, we can form the intersection A intersection B intersection C in two ways: (A intersection B) intersection C and A intersection (B intersection C) Prove that it is possible to define a function g P(U) times P(U) times P(U) rightarrow P(U), (A, B, C) rightarrow A intersection B intersection c regardless of the way of placing the brackets.
