MATH136 Lecture Notes - Identity Matrix, Row Echelon Form, Mathematical Induction

• School: University of Waterloo
• Department: Mathematics
• Course: MATH136
• Professor: Robert Sproule

Document Summary

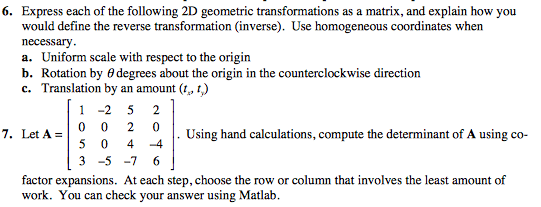
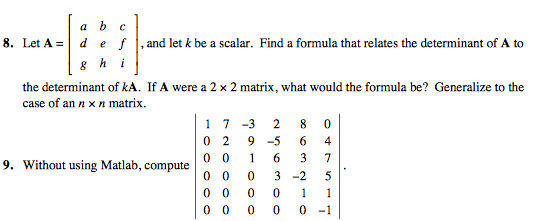
Monday, march 17 lecture 28 : determinants. 28. 1 determinants let m denote the set of all square matrices. A determinant can be viewed as a function d : m which maps any n n matrix a to a real number according to well defined formula applied to the entries of the matrix. Its general formula will be stated explicitly below. We denote the determinant function by det(a) or | a |. In the case of a 2 2 matrix a = [aij]2 2, the function det is defined as: det(a) = a11a22 a12a21. 28. 2 definition let a = [aij] be an n n matrix. We will refer to mij as a minor matrix of a associated to aij. We will call the number the minor of the element aij, or the (i, j) minor. mij = det(mij) = | mij | Remark note that mij is a matrix while mij is a real number.